In grade 8 in Ontario histograms are one of the new data management topics. Below is a series of activities that range from paper & pencil to virtual manipulatives. They start by a simple comparison of bar graphs and histograms to identify characteristics, then moves to some consolidation of ideas using a Desmos Sort. Then some data collection of student heights to formally introduce creating a histogram followed by some practice making histograms by hand and then with Google Sheets. If you did everything here it should take about 2-3 classes.
In grade 8 in Ontario histograms are one of the new data management topics. Below is a series of activities that range from paper & pencil to virtual manipulatives. They start by a simple comparison of bar graphs and histograms to identify characteristics, then moves to some consolidation of ideas using a Desmos Sort. Then some data collection of student heights to formally introduce creating a histogram followed by some practice making histograms by hand and then with Google Sheets. If you did everything here it should take about 2-3 classes.Grade 8
- collect and organize categorical, discrete, or continuous primary data and secondary data (e.g., electronic data from websites such as E-Stat or Census At Schools), and display the data in charts, tables, and graphs (including histograms and scatter plots) that have appropriate titles, labels (e.g., appropriate units marked on the axes), and scales (e.g., with appropriate increments) that suit the range and distribution of the data, using a variety of tools (e.g., graph paper, spreadsheets, dynamic statistical software);
- select an appropriate type of graph to represent a set of data, graph the data using technology, and justify the choice of graph (i.e., from types of graphs already studied, including histograms and scatter plots);
- read, interpret, and draw conclusions from primary data (e.g., survey results, measurements, observations) and from secondary data (e.g., election data or temperature data from the newspaper, data from the Internet about lifestyles), presented in charts, tables, and graphs (including frequency tables with intervals, histograms, and scatter plots);
- demonstrate an understanding of the appropriate uses of bar graphs and histograms by comparing their characteristics
- Could also be used as a review in MBF3C or MDM4U

- If you are doing the physical card sort then print graphs to card stock (we suggest each set is a different colour so that if students mix them up they are easy to separate). We also suggest lamination. There are two pages for a total of 16 cards.
- If you are doing any of the online activities then chromebooks/laptops/computers/iPads will be needed. You will also need to make copies of the Desmos version of the card sort and/or the Desmos card sort consolidation.
- Copies of the handout.

- The purpose of the card sort is to start to distinguish both histograms and bar graphs and continuous vs categorical data. But first have them do an open sort. Hand out cards to students (or give students the code for the Desmos Sort). Ask them to sort them in any way they wish. The only stipulations are that there should be at least two groups and each group must have at least two cards. Students will sort them in all kids of ways (by the numbers, by the topics, by the looks). Circulate and encourage them to explain how they were sorted.
- Once sorted it is likely that most will not have them sorted how you wish. Have them describe their sorts then ask them to sort them in a way so that there are only two groups.
- By this time some may have them sorted into bar graphs and histograms. If not show one bar graph and then one histogram stating that you want those cards to represent characteristics of each group (you can use the slideshow to show the graphs). Use these graphs to develop the difference between continuous and discrete data.
- Time to change the pace and have students collect some data. Have students measure their heights and put them on a dot plot on the board. Use this to create a histogram (without creating a tally). You might want to collect their heights in a spreadsheet so that you can create a histogram with it later.
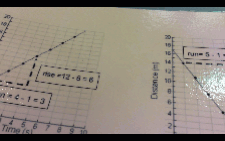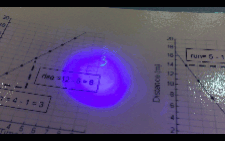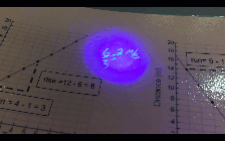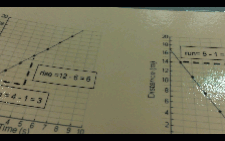
- Walk through the front of the handout to show how to create a histogram from data.
- Likely you will be at least done one class at this point (if not more). At the beginning of the second class, start with the consolidation Desmos card sort (with some stuff on average) to remind students of continuous and discrete data. Another possible way to start is to have students use this Google form to enter some examples of continuous and discrete data. You can take up the results.
- Once done taking up the worksheet you can then introduce students to creating histograms using Google Sheets. Share this sheet with continuous data. This video will tell you how.
- Card Sort Google Docs, PDF, Desmos Slide show of each graph
- Desmos Consolidation
- Handout Google Docs, PDF
- Handout Answers Google Docs, PDF
- Discrete/Continuous Form
- Spreadsheet Graphs, Answers